Maurits Cornelis Escher

1992/02/01 Angulo, Patxi Iturria: Elhuyar aldizkaria

• Matematika

M. C. Escher's life

March 27 marks the 20th anniversary of the death of Dutch illustrator Maurits Cornelis Escher. Escher was born 74 years earlier in the Dutch town of Leeuward.

In 1903 his family moved to the town of Arnheim, where he went to high school. Except for drawing, the rest of the subjects were desperate (Figure 1). In 1919 he began his studies at the Haarlem School of Architecture and Decorative Arts. But soon after he left and began to study graphic arts taught at the same school, with Samuel Jesserum of Mesquita as teacher. Two years later he left the School of Art, mastering the basic knowledge of drawing and drawing in wood.

In 1922 he travelled through Italy and Spain. After spending the winter in Siena he moved to the south of Italy, specifically to the village of Ravello. There he met Jetta Umiker, his wife. After getting married they moved to Rome, Monte Verde (Figure 2). For the first time I would work calmly. Until 1935 he lived as at home in Italy. He travelled spring to the mountains of Abruzzi, Campania, Corsica, Malta, Sicily, etc., usually with other painters (Giuseppe Haas Triverio, Robert Schiess, etc. ). In these trips Escher realized his emotions (which he would later use in his drawings). In 1929 Castrovalvan represented a landscape without borders (Castrovalva, 1929), one of the first to extol Escher, one of the best realized by him.

Escher was until then quite unknown. A couple of small exhibitions, several books and kitto. He did not sell his works and lived from his father's back. Until 1951 he could not live from his work.

From 1935 the Italian atmosphere became dark due to fascism. From there they went to the Swiss village of Châteaux-d´Oex, where he assured that two winters lived in “white nostalgia”. The environment, the landscape, the architecture, etc. did not suggest anything. Leaving there became obsession. He had marine nostalgia. For this reason, he sent a letter to the “Compagnia Adria” of Fiume, a company that organized maritime trips through the Mediterranean, asking if they had a free place. Escher's proposal was as follows: The Jett and its passage, with drawings based on the emotions made during the trip, would pay with 48 images (4 copies of 12 plates).

More surprising was the affirmative response. In 1936 they traveled Fiume-Valencia and Genoa-Valencia. Of that time belong, among others, the drawings Idi-begia and Marseille. Taking advantage of these trips he visited the Alhambra of Granada with Jett. He studied his ornaments in depth and on his second visit was very copied. This laid the foundation for his pioneering work in the field of periodic surface partitions. He was imprisoned in Cartagena for copying the defensive facilities of the city, ancient walls. All his images were confiscated.

In 1937 they moved to the Dutch village of Baarn, where high school had great prestige. The production of the time is abundant, since its climate favored well. Thereafter his life relaxed. Despite having made more trips around the Mediterranean, he did not get old suggestions (Figure 3). Only when he became ill in 1962, he stopped imagining. In 1969 Snake made the drawing, showing that he was still in good shape.

In 1970 he moved to the Rosa Spier house in Laren (Holland). In that house the artists had their studies and they lacked nothing. He died there two years later.

M. C. Work of Escher

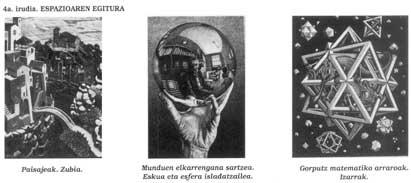
In Escher's works, except in the landscapes made before 1937, we can find a mathematical suggestion. It seems that in his 70 images he explains his research. In these studies he deals with three areas of mathematics (Figure 4).

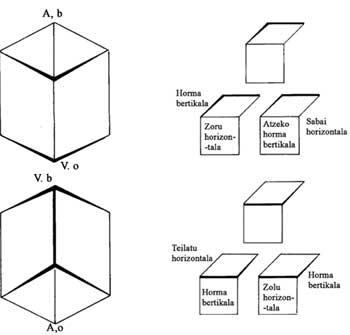
Space structure

Throughout his work he exposed this concern. Since 1937 his paintings collected with great logic the different spaces. His interest in mere mathematical image came later, created by crystals. It is therefore an area with landscapes, rare worlds and mathematical bodies that penetrate each other.

Surface structure

This period began when he took care of the regular partition of the surface, especially influenced by the visit to the Alhambra of Granada. After a thorough analysis he invented a method for the regular distribution of the smooth surface. There is no picture with just this theme. However, Escher used these partitions in images of metamorphosis in which simple mathematical images become people, plants, animals, etc. Also in the images of the cycles, in which the final image coincides with the initial one, this partition was used. Finally, this technique also appears in approaches to infinity, but in them it used uniform images. The difficulty of this work explains why they appeared later.

Projection of the flat surface of the three-dimensional space

Escher immediately encountered the problems of representation in two dimensions of three-dimensional images. He rigorously studied the laws of perspective that were known since the Renaissance and discovered new laws that explained them in several images. The image is the projection on the launa surface of a three-dimensional object, existing or not in space. We can find three types of pictures: those that present the problem of imagination, those that speak of perspective and those that have impossible images.

These three areas did not differ throughout life. However, he often analyzed several topics at once.

Landscapes occupy the first epoch (1922-37), especially in southern Italy and on the Mediterranean coast. The most complete lithograph is Hiru mundu (1955). Already in the lithograph Natura hila of 1934 we can see the interpenetration of two worlds through a mirror.

The 1937-45 season is the epoch of metamorphosis, when the 1937 Metamorphosis begins with Table I. To cite a work of this time, we call Day and Night (1938). In it you can see all the characteristics of the time. At the same time, metamorphosis, a cycle and the change of two-dimensional to three-dimensional images are presented. The last work of this time dates back to 1946, the Magic Mirror. The work that best explains the change between two and three dimensions is The Hands Represented, 1948, and the last Dragon, 1952.

The subject of perspective had already worked on the tower of Babel in 1928 and the tower of St. Peter in 1935, Rome. It highlights that what interested Escher was not one thing, but the same perspective. However, until 1946 the laws were not deeply studied. Another world of 1946 is a stamp point I, nadir and escape point at the same time. The summit of the time is reached without lips in Goiko eta behetik (1947). The picture has parallel lines that cut together. At the end of the period he returned to the traditional perspective and dealt with the basic geometric figures (polyhedra, spirals, Möbius tapes,...). The first work is the Crystal of 1947, at the top we have the Stars (1948) and the last is the tetrahedral Planetoid of 1954. They also appear in later works but not as the main topic. Möbius tapes are included in this group even later.

With the work El cada vez menor I of 1956 begins the phase of approach to infinity. Escher himself considers that the best work is the Circular Limit III (1959). Escher's last work, Snakes (1969), is also an approximation. This time also includes impossible figures. The first is Concave and Convex (1955) and the last Waterfall (1961). The most surprising work of the time, and one of the most important of all Escher's work, is the Drawing Room (1956). According to Escher, this work reached the limit of its intellectual and artistic capacity.

Escher's work underwent a sharp change between 1934 and 1937. This change has to do with the transfer of Rome and the Mediterranean to Switzerland, the Netherlands and Belgium. However, change would not have been possible if in Escher this attitude had not existed before. This attitude should not be sought in his special interest in science or mathematics. Escher always said that he did not know about geometry. In an interview he said: “In mathematics I got nothing.

It seems curious that, without realizing it, he spoke of mathematics. No, at school I was a charming and naive boy. Who thought that mathematicians would decorate my books with images and that, with such expert people, I would be treated like my classmates or siblings? And they can’t believe that I don’t understand anything they say.” He was not a mathematician and, above all, Escher was an artisan who worked with the metro and compass to achieve a specific goal.

In the initial works this interest is already perceived. In 1920 he made in the Cathedral of St. Bavon of Haarlem a candlestick on a sheet larger than a meter feather, under which in a ball was reflected the cathedral and the craftsman himself. Another 1922 drawing presents a surface full of heads, with four of them served. All cover the surface. In 1937, after the second visit to the Alhambra, a succession of paintings immediately appeared.

G in 1938. H.´s-Gravesande dedicated an article in the magazine “Elseviers Maandschrift”. However, until 1951 official artistic criticism had forgotten it. In February 1951 an article by Marc Severin appeared in the magazine “The Studio”. According to Severin, Escher knew how to bring to light the mathematical poetry that keeps things. In the magazine “Jardin des Arts” of October 1965, Albert Flocon said: “Concepts such as high and low, interior and exterior, left and right, near and far seem to lose all absolute sense, becoming interchangeable. On the other hand, suddenly, between points, surfaces and spaces, between causes and consequences, we focus on new ties, creating before our eyes new spatial structures that conjure rare worlds and are as possible as ours”.

In 1968, on the occasion of Escher's 70th anniversary, the Hague Museum organised a large exhibition. The Dutch Foreign Minister commissioned a film that ended in 1970. Jurriaan Andriessen composed in 1970 the piece suggested by the images of Escher. He played the Rotterdam Philharmonic Orchestra while the images of Escher were seen. Currently Escher is one of the best-known graphic artists.

(Note: To see the text of this photo, go to pdf).